Eight Standard >> Exponents or power or index | Part -1

Product law of exponents

 We can write 
\(2 \times 2 \times 2 \)=2^{3}\)
\(2 \times 2 \times 2 \times 2\)=2^{4}\)
\(2 \times 2 \times 2 ......n times\)=2^{n}\)

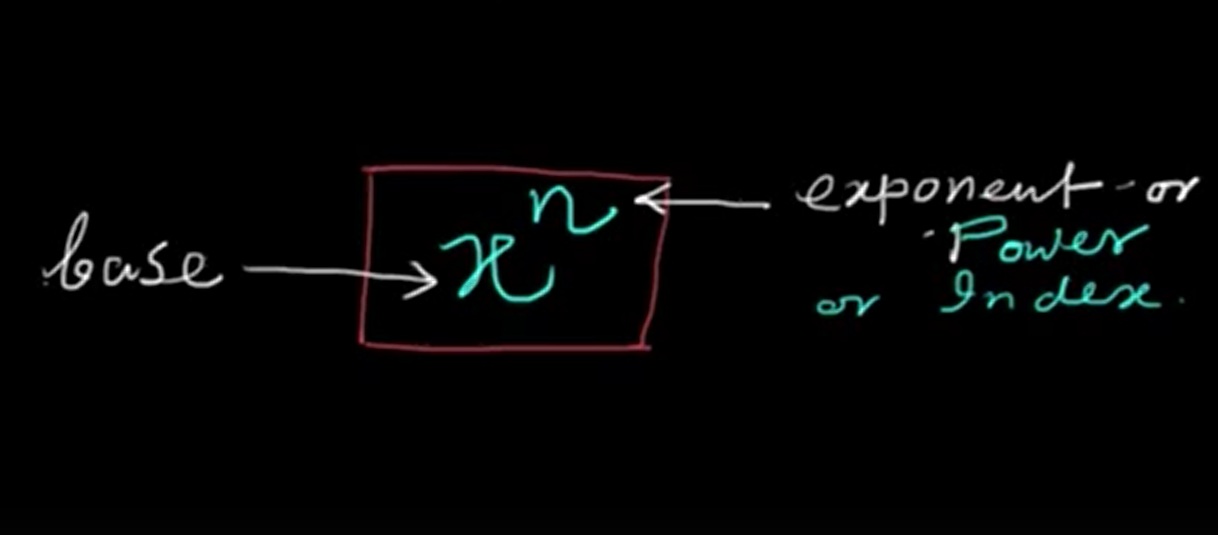
Rule 1. If \frac{p}{q} is any rational number then for any positive integer n we have \(\left(\frac{p}{q}\right)^{n}=\frac{p^{n}}{q^{n}}\)

Ex:  \(\left(\frac{2}{3}\right)^{4}=\frac{2^{4}}{3^{4}}=\frac{16}{81}\)
\(\left(-\frac{4}{5}\right)^{3}=\frac{(-4) \times (-4) \times (-4)}{5 \times 5 \times 5}=\frac{-64}{125}\)

Rule 2. For a rational number \(\frac{p}{q}\), where \(p \neq 0,\ q \neq 0,\) we have \(\left(\frac{p}{q}\right)^{-1}=\frac{q}{p}\) [it is reciprocal or inverse of \(\frac{p}{q}\)]

 \(\left(\frac{p}{q}\right)^{-n}=\left(\frac{q}{p}\right)^{n}\)

Ex: \(\left(-\frac{2}{3}\right)^{-3}=\left(-\frac{3}{2}\right)^{3}\)
      \(\left(\frac{2}{5}\right)^{-1}=\left(\frac{5}{2}\right)\)